What Part Of The Atom Undergoes Change During Radioactive Decay
3.1: Nuclear Chemistry and Radioactive Decay
- Folio ID
- 98691
Skills to Develop
- Draw nuclear structure in terms of protons, neutrons, and electrons
- Recognize the role of mass defect and binding energy for nuclei in atomic masses
- Explain trends in the relative stability of nuclei
- Place common particles and energies involved in nuclear reactions
- Write and remainder nuclear equations
- Recognize mutual modes of radioactive decay
- Describe kinetic parameters for decay processes, including half-life
- Describe mutual radiometric dating techniques
A Review of Isotopes and Introduction to Radioactivity
Video \(\PageIndex{1}\): A video review of isotopes (Unit 2), with a peek at radioactive isotopes.
Nuclear Chemical science - An Introduction
Nuclear chemistry is the study of reactions that involve changes in nuclear structure. The chapter on atoms, molecules, and ions introduced the basic idea of nuclear structure, that the nucleus of an atom is equanimous of protons and, with the exception of \(\ce{^1_1H}\), neutrons. Recall that the number of protons in the nucleus is called the atomic number (\(Z\)) of the element, and the sum of the number of protons and the number of neutrons is the mass number (\(A\)). Atoms with the same atomic number but dissimilar mass numbers are isotopes of the aforementioned element. When referring to a single blazon of nucleus, nosotros often utilize the term nuclide and identify it by the notation:
\[\ce{^{A}_{Z}X} \characterization{Eq1}\]
where
- \(X\) is the symbol for the chemical element,
- \(A\) is the mass number, and
- \(Z\) is the diminutive number.
Frequently a nuclide is referenced by the name of the element followed past a hyphen and the mass number. For instance, \(\ce{^{14}_6C}\) is called "carbon-14."
Protons and neutrons, collectively called nucleons, are packed together tightly in a nucleus. With a radius of about x−15 meters, a nucleus is quite small compared to the radius of the unabridged cantlet, which is about x−x meters. Nuclei are extremely dumbo compared to majority matter, averaging \(1.8 \times ten^{fourteen}\) grams per cubic centimeter. For case, h2o has a density of 1 gram per cubic centimeter, and iridium, one of the densest elements known, has a density of 22.6 1000/cm3. If the earth'due south density were equal to the average nuclear density, the earth'southward radius would be but about 200 meters (earth's actual radius is approximately \(6.4 \times x^half dozen\) meters, xxx,000 times larger).
Recall our exploration of atomic size with this video:
Video \(\PageIndex{2}\): An exploration of atomic size. If this video looks familiar its considering we also saw information technology dorsum in Unit 2!
To hold positively charged protons together in the very small volume of a nucleus requires very potent attractive forces because the positively charged protons repel one some other strongly at such brusque distances. The strength of attraction that holds the nucleus together is the strong nuclear force. (The strong forcefulness is ane of the four primal forces that are known to be. The others are the electromagnetic forcefulness, the gravitational force, and the nuclear weak force.) This force acts between protons, between neutrons, and between protons and neutrons. Information technology is very unlike from the electrostatic force that holds negatively charged electrons effectually a positively charged nucleus (the attraction betwixt opposite charges). Over distances less than ten−xv meters and within the nucleus, the strong nuclear strength is much stronger than electrostatic repulsions between protons; over larger distances and outside the nucleus, it is essentially nonexistent.
Nuclear Bounden Energy
Every bit a simple example of the free energy associated with the strong nuclear strength, consider the helium atom composed of ii protons, ii neutrons, and two electrons. The full mass of these six subatomic particles may be calculated equally:
\[ \underset{\Large\text{protons}}{(2 \times 1.0073\; \text{amu})} + \underset{\Large\text{neutrons}}{(ii \times ane.0087\; \text{amu})} + \underset{\Large\text{electrons}}{(2 \times 0.00055\; \text{amu})}= 4.0331\; \text{amu }\label{Eq2}\]
Still, mass spectrometric measurements reveal that the mass of an \(\ce{_2^iv He}\) cantlet is 4.0026 amu, less than the combined masses of its six constituent subatomic particles. This difference betwixt the calculated and experimentally measured masses is known every bit the mass defect of the atom. In the case of helium, the mass defect indicates a "loss" in mass of 4.0331 amu – 4.0026 amu = 0.0305 amu. The loss in mass accompanying the formation of an atom from protons, neutrons, and electrons is due to the conversion of that mass into free energy that is evolved as the atom forms. The nuclear binding energy is the energy produced when the atoms' nucleons are bound together; this is also the free energy needed to interruption a nucleus into its constituent protons and neutrons. In comparison to chemic bond energies, nuclear binding energies are vastly greater, every bit we volition learn in this department. Consequently, the free energy changes associated with nuclear reactions are vastly greater than are those for chemical reactions.
The conversion betwixt mass and energy is most identifiably represented by the mass-energy equivalence equation as stated by Albert Einstein:
\[Due east=mc^2 \label{Eq3}\]
where Eastward is energy, m is mass of the matter being converted, and c is the speed of lite in a vacuum. This equation tin can be used to find the amount of free energy that results when matter is converted into energy. Using this mass-energy equivalence equation, the nuclear binding energy of a nucleus may be calculated from its mass defect, a adding across the telescopic of our course. A variety of units are commonly used for nuclear binding energies, including electron volts (eV), with 1 eV equaling the amount of energy necessary to the move the charge of an electron across an electric potential deviation of 1 volt, making \(\mathrm{i\; eV = one.602 \times 10^{-19}\; J}\).
Because the free energy changes for breaking and forming bonds are so small compared to the energy changes for breaking or forming nuclei, the changes in mass during all ordinary chemical reactions are virtually undetectable. Equally we will discuss later in our unit of measurement on thermochemistry, the most energetic chemical reactions showroom enthalpies on the society of thousands of kJ/mol, which is equivalent to mass differences in the nanogram range (10–9 thousand). On the other hand, nuclear binding energies are typically on the order of billions of kJ/mol, corresponding to mass differences in the milligram range (10–3 g).
Nuclear Stability
A nucleus is stable if it cannot be transformed into another configuration without calculation energy from the outside. Of the thousands of nuclides that exist, about 250 are stable. A plot of the number of neutrons versus the number of protons for stable nuclei reveals that the stable isotopes fall into a narrow band. This region is known as the ring of stability (also called the belt, zone, or valley of stability). The straight line in Effigy \(\PageIndex{1}\) represents nuclei that accept a one:1 ratio of protons to neutrons (n:p ratio). Note that the lighter stable nuclei, in general, accept equal numbers of protons and neutrons. For example, nitrogen-fourteen has seven protons and vii neutrons. Heavier stable nuclei, yet, have increasingly more neutrons than protons. For case: iron-56 has 30 neutrons and 26 protons, an northward:p ratio of i.xv, whereas the stable nuclide lead-207 has 125 neutrons and 82 protons, an n:p ratio equal to 1.52. This is because larger nuclei take more proton-proton repulsions, and crave larger numbers of neutrons to provide compensating strong forces to overcome these electrostatic repulsions and agree the nucleus together.
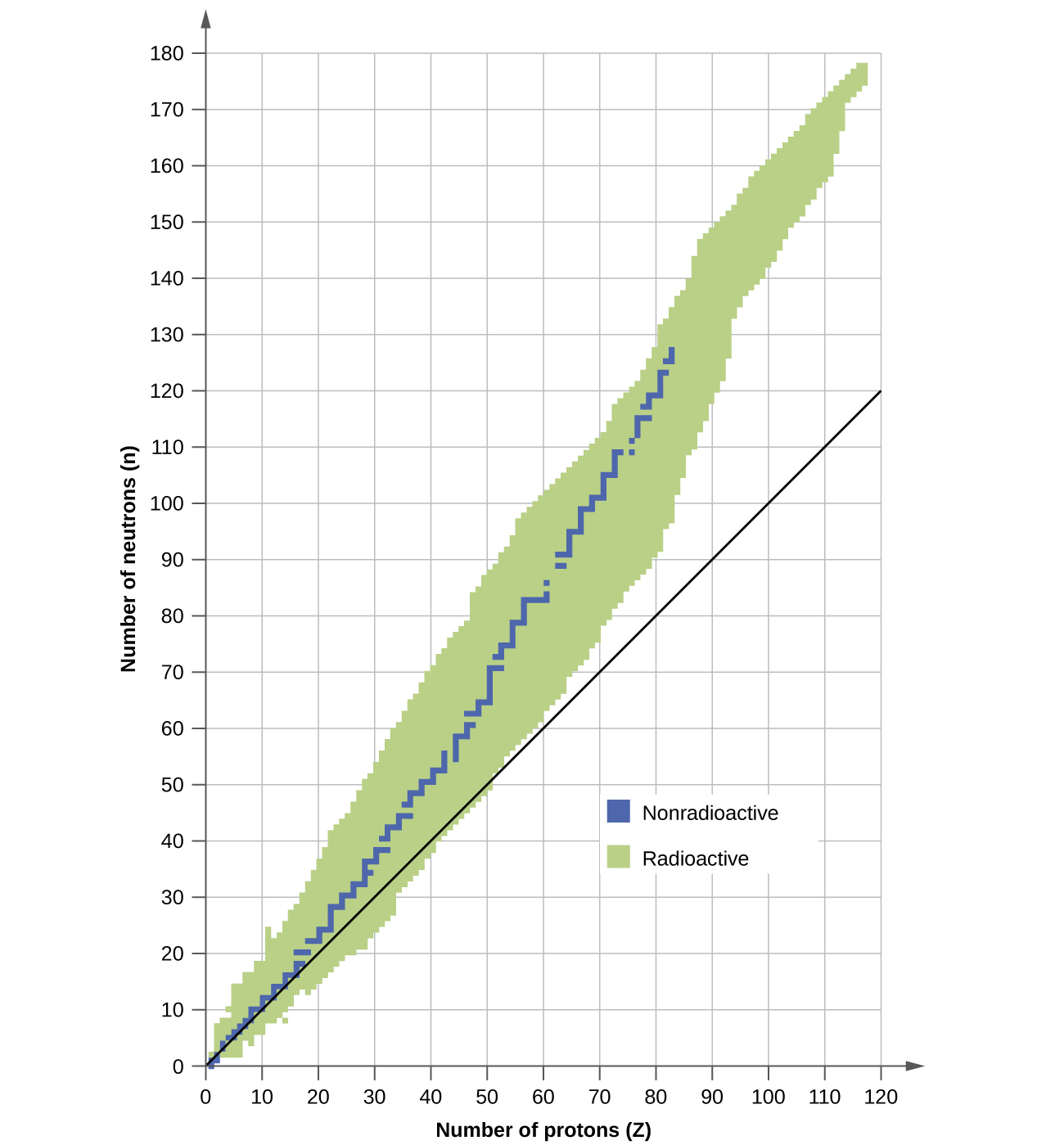
Figure \(\PageIndex{1}\): This plot shows the nuclides that are known to exist and those that are stable. The stable nuclides are indicated in blue, and the unstable nuclides are indicated in green. Note that all isotopes of elements with diminutive numbers greater than 83 are unstable. The solid line is the line where north = Z.
The nuclei that are to the left or to the right of the band of stability are unstable and exhibit radioactivity. They change spontaneously (disuse) into other nuclei that are either in, or closer to, the band of stability. These nuclear decay reactions convert one unstable isotope (or radioisotope) into another, more stable, isotope. Nosotros volition discuss the nature and products of this radioactive decay in subsequent sections of this unit.
Several observations may be made regarding the relationship between the stability of a nucleus and its structure. Nuclei with even numbers of protons, neutrons, or both are more likely to be stable (Table \(\PageIndex{1}\)). Nuclei with certain numbers of nucleons, known as magic numbers , are stable against nuclear decay. These numbers of protons or neutrons (two, viii, twenty, 28, fifty, 82, and 126) make consummate shells in the nucleus. These are like in concept to the stable electron shells observed for the noble gases. Nuclei that have magic numbers of both protons and neutrons, such as \(\ce{^4_2He}\), \(\ce{^{xvi}_8O}\), \(\ce{^{40}_{twenty}Ca}\), and \(\ce{^{208}_{82}Pb}\) and are specially stable. These trends in nuclear stability may exist rationalized by considering a quantum mechanical model of nuclear free energy states analogous to that used to depict electronic states, which we will discuss later in this course. The details of this model are across the telescopic of this course.
| Number of Stable Isotopes | Proton Number | Neutron Number |
|---|---|---|
| 157 | even | even |
| 53 | even | odd |
| 50 | odd | even |
| five | odd | odd |
The relative stability of a nucleus is correlated with its binding free energy per nucleon, the total bounden energy for the nucleus divided by the number or nucleons in the nucleus. For instance, the binding energy for a \(\ce{^4_2He}\) nucleus is therefore:
\[\mathrm{\dfrac{28.4\; MeV}{4\; nucleons}=seven.ten\; MeV/nucleon} \label{Eq3a}\]
The binding energy per nucleon of a nuclide on the curve shown in Figure \(\PageIndex{2}\)
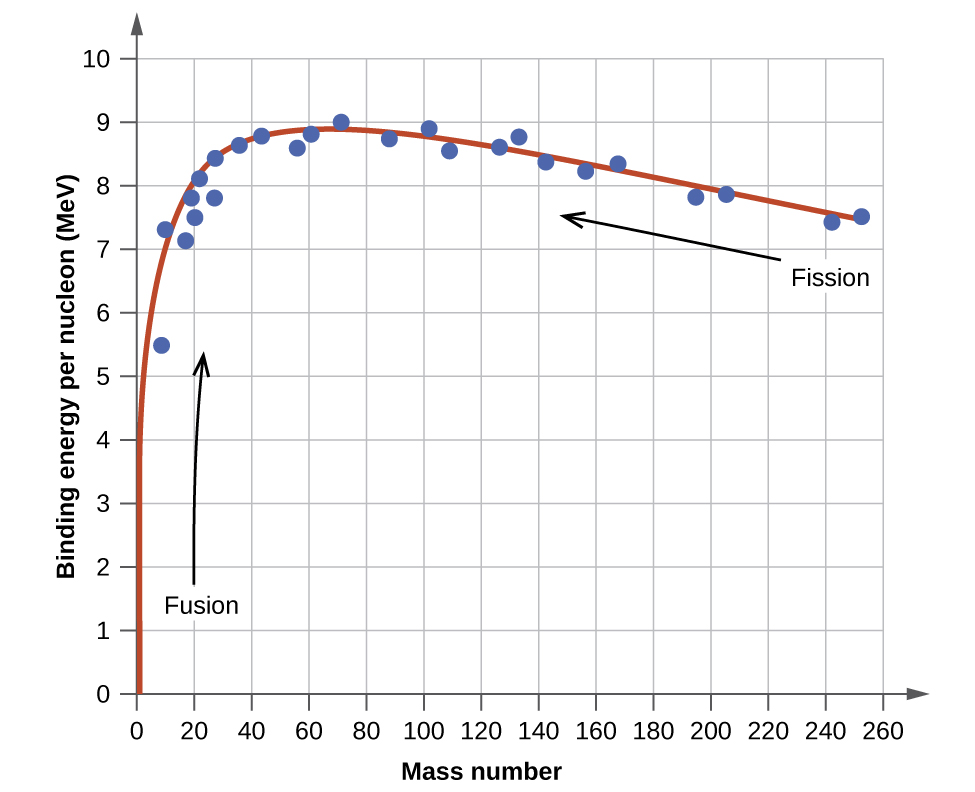
Effigy \(\PageIndex{2}\): The bounden energy per nucleon is largest for nuclides with mass number of approximately 56.
Changes of nuclei that result in changes in their atomic numbers, mass numbers, or free energy states are nuclear reactions . To describe a nuclear reaction, we use an equation that identifies the nuclides involved in the reaction, their mass numbers and atomic numbers, and the other particles involved in the reaction.
Types of Particles in Nuclear Reactions
Video \(\PageIndex{iii}\): A brief overview of the different types of radioactive decay.
Many entities tin be involved in nuclear reactions. The near mutual are protons, neutrons, alpha particles, beta particles, positrons, and gamma rays, as shown in Effigy \(\PageIndex{3}\). Protons
Figure \(\PageIndex{3}\): Although many species are encountered in nuclear reactions, this table summarizes the names, symbols, representations, and descriptions of the nigh common of these.
Note that positrons are exactly similar electrons, except they have the opposite charge. They are the most mutual example of antimatter, particles with the same mass but the contrary country of another belongings (for case, accuse) than ordinary matter. When antimatter encounters ordinary matter, both are annihilated and their mass is converted into free energy in the form of gamma rays (γ)—and other much smaller subnuclear particles, which are beyond the scope of this affiliate—co-ordinate to the mass-energy equivalence equation \(E = mc^2\), seen in the preceding section. For example, when a positron and an electron collide, both are annihilated and two gamma ray photons are created:
\[\ce{^0_{−1}e + ^0_{+one}e } \rightarrow \gamma + \gamma \characterization{21.3.i}\]
Gamma rays compose short wavelength, high-free energy electromagnetic radiation and are (much) more than energetic than better-known Ten-rays. Gamma rays are produced when a nucleus undergoes a transition from a college to a lower energy state, similar to how a photon is produced past an electronic transition from a higher to a lower energy level. Due to the much larger free energy differences between nuclear energy shells, gamma rays emanating from a nucleus take energies that are typically millions of times larger than electromagnetic radiations emanating from electronic transitions.
Balancing Nuclear Reactions
A balanced chemic reaction equation reflects the fact that during a chemical reaction, bonds break and form, and atoms are rearranged, but the total numbers of atoms of each element are conserved and practice not change. A counterbalanced nuclear reaction equation indicates that at that place is a rearrangement during a nuclear reaction, but of subatomic particles rather than atoms. Nuclear reactions also follow conservation laws, and they are balanced in two means:
- The sum of the mass numbers of the reactants equals the sum of the mass numbers of the products.
- The sum of the charges of the reactants equals the sum of the charges of the products.
If the atomic number and the mass number of all simply i of the particles in a nuclear reaction are known, nosotros tin identify the particle by balancing the reaction. For example, we could determine that
Example \(\PageIndex{1}\): Balancing Equations for Nuclear Reactions
The reaction of an α particle with magnesium-25
Solution
The nuclear reaction tin exist written as:
\[\ce{^{25}_{12}Mg + ^4_2He \rightarrow ^1_1H + ^{A}_{Z}X}\]
where
- \(\ce A\) is the mass number and
- \(\ce Z\) is the diminutive number of the new nuclide, \(\ce X\).
Because the sum of the mass numbers of the reactants must equal the sum of the mass numbers of the products:
\[\mathrm{25+iv=A+1}\]
so
\[ \mathrm{A=28}\]
Similarly, the charges must balance, so:
\[\mathrm{12+2=Z+1}\]
and so
\[\mathrm{Z=13}\]
Cheque the periodic table: The chemical element with nuclear charge = +xiii is aluminum. Thus, the product is
Exercise \(\PageIndex{ane}\)
The nuclide \(\ce{^{125}_{53}I}\) combines with an electron and produces a new nucleus and no other massive particles. What is the equation for this reaction?
- Reply:
-
\[\ce{^{125}_{53}I + ^0_{−i}e \rightarrow ^{125}_{52}Te} \nonumber\]
Following are the equations of several nuclear reactions that have of import roles in the history of nuclear chemistry:
- The first naturally occurring unstable element that was isolated, polonium, was discovered by the Smooth scientist Marie Curie and her hubby Pierre in 1898. It decays, emitting α particles: \[\ce{^{212}_{84}Po⟶ ^{208}_{82}Pb + ^4_2He}\]
- The beginning nuclide to be prepared past artificial means was an isotope of oxygen, 17O. It was made past Ernest Rutherford in 1919 past bombarding nitrogen atoms with α particles: \[\ce{^{14}_7N + ^4_2α⟶ ^{17}_8O + ^1_1H}\]
- James Chadwick discovered the neutron in 1932, every bit a previously unknown neutral particle produced forth with 12C past the nuclear reaction between 9Exist and 4He: \[\ce{^9_4Be + ^4_2He⟶ ^{12}_6C + ^1_0n}\]
- The showtime chemical element to exist prepared that does not occur naturally on the world, technetium, was created by bombardment of molybdenum past deuterons (heavy hydrogen, \(\ce{^2_1H}\)
) , past Emilio Segre and Carlo Perrier in 1937: \[ \ce{^2_1H + ^{97}_{42}Mo⟶ii^1_0n + ^{97}_{43}Tc}\] - The kickoff controlled nuclear concatenation reaction was carried out in a reactor at the University of Chicago in 1942. Ane of the many reactions involved was: \[ \ce{^{235}_{92}U + ^1_0n⟶ ^{87}_{35}Br + ^{146}_{57}La + 3^1_0n}\]
Post-obit the somewhat serendipitous discovery of radioactivity by Becquerel, many prominent scientists began to investigate this new, intriguing miracle. Among them were Marie Curie (Video \(\PageIndex{4}\); the showtime woman to win a Nobel Prize, and the only person to win two Nobel Prizes in different sciences—chemistry and physics), who was the first to coin the term "radioactivity," and Ernest Rutherford (of gold foil experiment fame), who investigated and named three of the most common types of radiation. During the beginning of the twentieth century, many radioactive substances were discovered, the properties of radiation were investigated and quantified, and a solid understanding of radiation and nuclear decay was developed.
Learn More about Marie Curie'south Life & Work
Video \(\PageIndex{4}\): A brief overview of the life and work of Marie Curie.
The spontaneous change of an unstable nuclide into some other is radioactive decay. The unstable nuclide is chosen the parent nuclide; the nuclide that results from the decay is known every bit the daughter nuclide. The daughter nuclide may be stable, or it may decay itself. The radiations produced during radioactive decay is such that the girl nuclide lies closer to the band of stability than the parent nuclide, so the location of a nuclide relative to the band of stability can serve as a guide to the kind of decay information technology will undergo (Figure \(\PageIndex{four}\)).
Figure \(\PageIndex{four}\): A nucleus of uranium-238 (the parent nuclide) undergoes α decay to grade thorium-234 (the daughter nuclide). The blastoff particle removes two protons (dark-green) and two neutrons (gray) from the uranium-238 nucleus.
Although the radioactivity of a nucleus is besides small-scale to come across with the naked eye, we can indirectly view radioactivity in an environment called a deject chamber. Video \(\PageIndex{v}\) is an opportunity to larn about cloud chambers and to view an interesting Cloud Chamber Sit-in from the Jefferson Lab.
Video \(\PageIndex{v}\): How to Build a Cloud Sleeping room!
Types of Radioactive Decay
Ernest Rutherford's experiments involving the interaction of radiations with a magnetic or electric field (Figure \(\PageIndex{v}\)) helped him decide that one type of radiation consisted of positively charged and relatively massive α particles; a second type was fabricated up of negatively charged and much less massive β particles; and a third was uncharged electromagnetic waves, γ rays. We now know that α particles are high-free energy helium nuclei, β particles are high-free energy electrons, and γ radiations compose high-energy electromagnetic radiation. We allocate different types of radioactive decay by the radiation produced.
Effigy \(\PageIndex{v}\): Blastoff particles, which are attracted to the negative plate and deflected by a relatively minor amount, must be positively charged and relatively massive. Beta particles, which are attracted to the positive plate and deflected a relatively large corporeality, must be negatively charged and relatively light. Gamma rays, which are unaffected by the electrical field, must be uncharged.
Blastoff (α) decay is the emission of an α particle from the nucleus. For example, polonium-210 undergoes α decay:
\[\ce{^{210}_{84}Po⟶ ^4_2He + ^{206}_{82}Pb} \hspace{40px}\ce{or}\hspace{40px} \ce{^{210}_{84}Po ⟶ ^4_2α + ^{206}_{82}Pb}\]
Alpha disuse occurs primarily in heavy nuclei (A > 200, Z > 83). Considering the loss of an α particle gives a daughter nuclide with a mass number four units smaller and an diminutive number two units smaller than those of the parent nuclide, the daughter nuclide has a larger n:p ratio than the parent nuclide. If the parent nuclide undergoing α decay lies below the band of stability, the daughter nuclide will lie closer to the band.
Beta (β) decay is the emission of an electron from a nucleus. Iodine-131 is an instance of a nuclide that undergoes β decay:
\[\ce{^{131}_{53}I ⟶ ^0_{-1}e + ^{131}_{54}X} \hspace{40px}\ce{or}\hspace{40px} \ce{^{131}_{53}I ⟶ ^0_{-one}β + ^{131}_{54}Xe}\]
Beta decay, which can exist thought of as the conversion of a neutron into a proton and a β particle, is observed in nuclides with a large due north:p ratio. The beta particle (electron) emitted is from the atomic nucleus and is non 1 of the electrons surrounding the nucleus. Such nuclei lie above the band of stability. Emission of an electron does non change the mass number of the nuclide merely does increase the number of its protons and decrease the number of its neutrons. Consequently, the n:p ratio is decreased, and the daughter nuclide lies closer to the ring of stability than did the parent nuclide.
Gamma emission (γ emission) is observed when a nuclide is formed in an excited state and and so decays to its basis state with the emission of a γ ray, a quantum of high-free energy electromagnetic radiation. The presence of a nucleus in an excited state is often indicated past an asterisk (*). Cobalt-60 emits γ radiations and is used in many applications including cancer treatment:
\[\mathrm{^{60}_{27}Co^* ⟶\, ^0_0γ +\, ^{threescore}_{27}Co}\]
There is no change in mass number or atomic number during the emission of a γ ray unless the γ emission accompanies i of the other modes of decay.
Positron emission (β+ decay) is the emission of a positron from the nucleus. Oxygen-15 is an example of a nuclide that undergoes positron emission:
\[\ce{^{15}_8O ⟶ ^0_{+1}e + ^{xv}_7N} \hspace{40px}\ce{or}\hspace{40px} \ce{^{15}_8O ⟶ ^0_{+one}β + ^{15}_7N}\]
Positron emission is observed for nuclides in which the n:p ratio is low. These nuclides lie beneath the band of stability. Positron decay is the conversion of a proton into a neutron with the emission of a positron. The n:p ratio increases, and the daughter nuclide lies closer to the band of stability than did the parent nuclide.
Electron capture occurs when one of the inner electrons in an atom is captured by the atom's nucleus. For case, potassium-40 undergoes electron capture:
\[\ce{^{40}_{nineteen}Thousand + ^0_{-i}e ⟶ ^{40}_{18}Ar}\]
Electron capture occurs when an inner shell electron combines with a proton and is converted into a neutron. The loss of an inner shell electron leaves a vacancy that will exist filled by one of the outer electrons. As the outer electron drops into the vacancy, it volition emit energy. In most cases, the energy emitted will be in the form of an Ten-ray. Like positron emission, electron capture occurs for "proton-rich" nuclei that lie beneath the band of stability. Electron capture has the same consequence on the nucleus as does positron emission: The atomic number is decreased by i and the mass number does non modify. This increases the n:p ratio, and the daughter nuclide lies closer to the band of stability than did the parent nuclide. Whether electron capture or positron emission occurs is difficult to predict. The choice is primarily due to kinetic factors, with the one requiring the smaller activation energy beingness the one more probable to occur. Figure \(\PageIndex{3}\) summarizes these types of decay, along with their equations and changes in atomic and mass numbers.
Figure \(\PageIndex{vi}\): This table summarizes the type, nuclear equation, representation, and any changes in the mass or diminutive numbers for various types of decay.
PET Browse
Positron emission tomography (PET) scans use radiation to diagnose and track wellness conditions and monitor medical treatments by revealing how parts of a patient's torso function (Figure \(\PageIndex{vii}\)). To perform a PET scan, a positron-emitting radioisotope is produced in a cyclotron and then attached to a substance that is used past the office of the torso being investigated. This "tagged" compound, or radiotracer, is then put into the patient (injected via IV or breathed in equally a gas), and how it is used by the tissue reveals how that organ or other expanse of the body functions.
Effigy \(\PageIndex{7}\): A PET scanner (a) uses radiation to provide an paradigm of how office of a patient's body functions. The scans it produces tin exist used to image a healthy encephalon (b) or can be used for diagnosing medical conditions such as Alzheimer'due south disease (c). (credit a: modification of work by Jens Maus)</
For example, F-18 is produced past proton battery of 18O
Radioactive Disuse Serial
The naturally occurring radioactive isotopes of the heaviest elements fall into chains of successive disintegrations, or decays, and all the species in one concatenation constitute a radioactive family unit, or radioactive decay series. Three of these serial include most of the naturally radioactive elements of the periodic tabular array. They are the uranium series, the actinide serial, and the thorium series. The neptunium series is a fourth series, which is no longer significant on the earth because of the brusque one-half-lives of the species involved. Each series is characterized past a parent (first fellow member) that has a long half-life and a series of daughter nuclides that ultimately lead to a stable terminate-product (Figure \(\PageIndex{8}\))—that is, a nuclide on the band of stability (Figure \(\PageIndex{1}\)). In all three series, the end-production is a stable isotope of lead. The neptunium serial, previously idea to terminate with bismuth-209, terminates with thallium-205.
Figure \(\PageIndex{8}\): Uranium-238 undergoes a radioactive decay series consisting of 14 split up steps before producing stable lead-206. This serial consists of viii α decays and six β decays.
Radioactive Half-Lives
Radioactive decay follows first-social club kinetics. Since first-order reactions have already been covered in particular in the kinetics chapter, we volition now employ those concepts to nuclear disuse reactions. Each radioactive nuclide has a feature, constant half-life (t 1/2), the time required for half of the atoms in a sample to decay. An isotope's half-life allows usa to determine how long a sample of a useful isotope volition be available, and how long a sample of an undesirable or dangerous isotope must be stored earlier it decays to a low-enough radiation level that is no longer a trouble.
For example, cobalt-sixty, an isotope that emits gamma rays used to treat cancer, has a half-life of 5.27 years (Figure \(\PageIndex{6}\)). In a given cobalt-threescore source, since one-half of the
Effigy \(\PageIndex{9}\): For cobalt-60, which has a half-life of 5.27 years, l% remains afterward 5.27 years (one half-life), 25% remains after 10.54 years (2 half-lives), 12.5% remains subsequently 15.81 years (3 half-lives), and then on.
Since nuclear decay follows get-go-guild kinetics, nosotros can adapt the mathematical relationships used for first-lodge chemical reactions. We generally substitute the number of nuclei, N, for the concentration. If the rate is stated in nuclear decays per 2d, we refer to it as the activity of the radioactive sample. The rate for radioactive decay is:
\[\text{decay rate} = \lambda N\]
with \(\lambda\) is the decay constant for the particular radioisotope.
The decay constant, \(\lambda\), which is the same every bit a rate constant discussed in the kinetics chapter. It is possible to express the disuse constant in terms of the one-half-life, t i/2:
\[λ=\dfrac{\ln 2}{t_{1/2}}=\dfrac{0.693}{t_{1/2}} \hspace{40px}\ce{or}\hspace{40px} t_{1/ii}=\dfrac{\ln 2}{λ}=\dfrac{0.693}{λ}\]
The first-order equations relating amount, N, and time are:
\[N_t=N_0e^{−kt} \hspace{40px}\ce{or}\hspace{40px} t=−\dfrac{1}{λ}\ln\left(\dfrac{N_t}{N_0}\right)\]
where N 0 is the initial number of nuclei or moles of the isotope, and Nt is the number of nuclei/moles remaining at time t. We will not concern ourselves with the calculation of half-life in this form.
Because each nuclide has a specific number of nucleons, a item balance of repulsion and attraction, and its ain degree of stability, the one-half-lives of radioactive nuclides vary widely. For example: the half-life of
| Type | Decay Mode | Half-Life | Uses |
|---|---|---|---|
| F-18 | β+ decay | 110. minutes | PET scans |
| Co-lx | β decay, γ decay | v.27 years | cancer treatment |
| Tc-99m 1 | γ disuse | 8.01 hours | scans of encephalon, lung, heart, bone |
| I-131 | β decay | viii.02 days | thyroid scans and treatment |
| Tl-201 | electron capture | 73 hours | heart and arteries scans; cardiac stress tests |
| The "thousand" in Tc-99m stands for "metastable," indicating that this is an unstable, high-energy land of Tc-99. Metastable isotopes emit \(γ\) radiations to rid themselves of excess energy and get (more) stable. | |||
Radiometric Dating
Several radioisotopes accept half-lives and other properties that brand them useful for purposes of "dating" the origin of objects such as archaeological artifacts, formerly living organisms, or geological formations. This process is radiometric dating and has been responsible for many breakthrough scientific discoveries almost the geological history of the earth, the development of life, and the history of homo culture. We will explore some of the most common types of radioactive dating and how the particular isotopes work for each type.
Radioactive Dating Using Carbon-14
The radioactive decay of carbon-14 provides a method for dating objects that were a part of a living organism. This method of radiometric dating, which is besides called radiocarbon dating or carbon-14 dating, is accurate for dating carbon-containing substances that are up to about 30,000 years old, and can provide reasonably accurate dates up to a maximum of about fifty,000 years old.
Naturally occurring carbon consists of three isotopes:
\[\ce{^{14}_7N + ^1_0n⟶ ^{14}_6C + ^1_1H}\]
All isotopes of carbon react with oxygen to produce CO2 molecules. The ratio of
\[\ce{^{xiv}_6C⟶ ^{14}_7N + ^0_{-i}east}\]
Thus, the \(\ce{^{14}_6C: ^{12}_6C}\)
Effigy \(\PageIndex{x}\): Along with stable carbon-12, radioactive carbon-14 is taken in by plants and animals, and remains at a abiding level within them while they are alive. Afterwards death, the C-fourteen decays and the C-14:C-12 ratio in the remains decreases. Comparing this ratio to the C-14:C-12 ratio in living organisms allows us to determine how long ago the organism lived (and died).
For example, with the half-life of
In that location have been some significant, well-documented changes to the
Radioactive Dating Using Nuclides Other than Carbon-14
Radioactive dating can also employ other radioactive nuclides with longer half-lives to engagement older events. For example, uranium-238 (which decays in a series of steps into atomic number 82-206) can be used for establishing the historic period of rocks (and the approximate age of the oldest rocks on earth). Since U-238 has a half-life of 4.5 billion years, information technology takes that amount of time for half of the original U-238 to decay into Lead-206. In a sample of stone that does not contain appreciable amounts of Pb-208, the most abundant isotope of atomic number 82, we tin assume that pb was not nowadays when the rock was formed. Therefore, by measuring and analyzing the ratio of U-238:Pb-206, we tin can determine the age of the rock. This assumes that all of the lead-206 nowadays came from the decay of uranium-238. If there is additional pb-206 present, which is indicated by the presence of other lead isotopes in the sample, it is necessary to make an adjustment. Potassium-argon dating uses a like method. Thou-40 decays past positron emission and electron capture to grade Ar-xl with a one-half-life of ane.25 billion years. If a stone sample is crushed and the amount of Ar-40 gas that escapes is measured, determination of the Ar-xl:M-40 ratio yields the age of the rock. Other methods, such as rubidium-strontium dating (Rb-87 decays into Sr-87 with a half-life of 48.8 billion years), operate on the aforementioned principle. To estimate the lower limit for the earth's historic period, scientists determine the age of diverse rocks and minerals, making the assumption that the earth is older than the oldest rocks and minerals in its crust. As of 2014, the oldest known rocks on earth are the Jack Hills zircons from Australia, found by uranium-pb dating to be nearly 4.iv billion years old.
Summary
Video \(\PageIndex{6}\): A video summary of some of the nuclear chemistry you just learned about.
An atomic nucleus consists of protons and neutrons, collectively called nucleons. Although protons repel each other, the nucleus is held tightly together by a short-range, but very stiff, force called the strong nuclear forcefulness. A nucleus has less mass than the total mass of its constituent nucleons. This "missing" mass is the mass defect, which has been converted into the binding energy that holds the nucleus together co-ordinate to Einstein's mass-energy equivalence equation, East = mc 2. Of the many nuclides that be, only a small number are stable. Nuclides with even numbers of protons or neutrons, or those with magic numbers of nucleons, are peculiarly probable to exist stable. These stable nuclides occupy a narrow ring of stability on a graph of number of protons versus number of neutrons. The binding free energy per nucleon is largest for the elements with mass numbers nearly 56; these are the most stable nuclei.
Nuclei tin undergo reactions that change their number of protons, number of neutrons, or energy state. Many different particles can be involved in nuclear reactions. The most mutual are protons, neutrons, positrons (which are positively charged electrons), blastoff (α) particles (which are high-energy helium nuclei), beta (β) particles (which are high-energy electrons), and gamma (γ) rays (which compose high-free energy electromagnetic radiation). As with chemical reactions, nuclear reactions are always balanced. When a nuclear reaction occurs, the total mass (number) and the total charge remain unchanged.
Nuclei that accept unstable n:p ratios undergo spontaneous radioactive disuse. The most common types of radioactivity are α decay, β decay, γ emission, positron emission, and electron capture. Nuclear reactions also often involve γ rays, and some nuclei decay by electron capture. Each of these modes of decay leads to the formation of a new nucleus with a more stable due north:p ratio. Some substances undergo radioactivity serial, proceeding through multiple decays before ending in a stable isotope. All nuclear decay processes follow get-go-order kinetics, and each radioisotope has its own characteristic half-life, the fourth dimension that is required for half of its atoms to decay. Because of the large differences in stability among nuclides, there is a very wide range of half-lives of radioactive substances. Many of these substances take constitute useful applications in medical diagnosis and handling, determining the age of archaeological and geological objects, and more.
Key Equations
- E = mc two
- disuse rate = λN
- \(t_{ane/2}=\dfrac{\ln 2}{λ}=\dfrac{0.693}{λ}\)
Glossary
- alpha (α) decay
- loss of an alpha particle during radioactivity
- alpha particle
- (α or
\(\ce{^4_2He}\) or \(\ce{^4_2α}\)) high-free energy helium nucleus; a helium atom that has lost two electrons and contains two protons and two neutrons - antimatter
- particles with the same mass but contrary backdrop (such every bit charge) of ordinary particles
- band of stability
- (besides, belt of stability, zone of stability, or valley of stability) region of graph of number of protons versus number of neutrons containing stable (nonradioactive) nuclides
- beta (β) decay
- breakdown of a neutron into a proton, which remains in the nucleus, and an electron, which is emitted equally a beta particle
- beta particle
-
( \(β\) or \(\ce{^0_{-1}east}\)or \(\ce{^0_{-one}β}\)) high-energy electron - bounden energy per nucleon
- total binding energy for the nucleus divided by the number of nucleons in the nucleus
- daughter nuclide
- nuclide produced past the radioactive decay of another nuclide; may be stable or may disuse further
- electron capture
- combination of a core electron with a proton to yield a neutron inside the nucleus
- electron volt (eV)
- measurement unit of nuclear binding energies, with 1 eV equaling the amount energy due to the moving an electron across an electric potential difference of i volt
- gamma (γ) emission
- decay of an excited-state nuclide accompanied past emission of a gamma ray
- gamma ray
- (γ or
\(\ce{^0_0γ}\)) curt wavelength, loftier-free energy electromagnetic radiation that exhibits moving ridge-particle duality - one-half-life (t i/2)
- time required for one-half of the atoms in a radioactive sample to decay
- magic number
- nuclei with specific numbers of nucleons that are inside the band of stability
- mass defect
- difference betwixt the mass of an atom and the summed mass of its constituent subatomic particles (or the mass "lost" when nucleons are brought together to form a nucleus)
- mass-energy equivalence equation
- Albert Einstein's relationship showing that mass and energy are equivalent
- nuclear bounden free energy
- free energy lost when an atom'due south nucleons are bound together (or the free energy needed to pause a nucleus into its constituent protons and neutrons)
- nuclear chemical science
- study of the structure of atomic nuclei and processes that modify nuclear structure
- nuclear reaction
- change to a nucleus resulting in changes in the atomic number, mass number, or energy state
- nucleon
- collective term for protons and neutrons in a nucleus
- nuclide
- nucleus of a particular isotope
- parent nuclide
- unstable nuclide that changes spontaneously into another (daughter) nuclide
- positron
( \(\ce{^0_{+1}β}\) or\(\ce{^0_{+1}e}\)) - antiparticle to the electron; it has identical properties to an electron, except for having the opposite (positive) accuse
- positron emission
- (besides, β+ decay) conversion of a proton into a neutron, which remains in the nucleus, and a positron, which is emitted
- radioactivity
- spontaneous decay of an unstable nuclide into some other nuclide
- radioactive decay series
- chains of successive disintegrations (radioactive decays) that ultimately lead to a stable end-product
- radioactivity
- phenomenon exhibited by an unstable nucleon that spontaneously undergoes change into a nucleon that is more stable; an unstable nucleon is said to be radioactive
- radiocarbon dating
- highly accurate means of dating objects 30,000–fifty,000 years old that were derived from one time-living matter; achieved by calculating the ratio of
\(\ce{^{14}_6C : ^{12}_6C}\) in the object vs. the ratio of\(\ce{^{xiv}_6C : ^{12}_6C}\) in the present-day atmosphere
- radioisotope
- isotope that is unstable and undergoes conversion into a different, more stable isotope
- radiometric dating
- utilize of radioisotopes and their properties to date the formation of objects such as archeological artifacts, formerly living organisms, or geological formations
- strong nuclear forcefulness
- forcefulness of attraction between nucleons that holds a nucleus together
Contributors
-
Paul Flowers (University of North Carolina - Pembroke), Klaus Theopold (Academy of Delaware) and Richard Langley (Stephen F. Austin Land Academy) with contributing authors.Textbook content produced past OpenStax Higher is licensed under a Artistic Eatables Attribution License 4.0 license. Download for free at http://cnx.org/contents/85abf193-2bd...a7ac8df6@ix.110).
- Adelaide Clark, Oregon Institute of Technology
- Crash Course Chemistry: Crash Course is a partitioning of Complexly and videos are free to stream for educational purposes.
- TED-Ed's delivery to creating lessons worth sharing is an extension of TED's mission of spreading dandy ideas. Within TED-Ed'south growing library of TED-Ed animations, y'all will find carefully curated educational videos, many of which represent collaborations between talented educators and animators nominated through the TED-Ed website.
- Fuse School, Open Educational Resources free of accuse, under a Creative Eatables License: Attribution-NonCommercial CC BY-NC (View License Deed: https://creativecommons.org/licenses/past-nc/iv.0/)
Feedback
Accept feedback to give virtually this text? Click here.
Establish a typo and want extra credit? Click here.
Source: https://chem.libretexts.org/Courses/Oregon_Institute_of_Technology/OIT:_CHE_201_-_General_Chemistry_I_%28Anthony_and_Clark%29/Unit_3:_Nuclei_Ions_and_Molecules/3.1:_Nuclear_Chemistry_and_Radioactive_Decay
Posted by: kerrseallegaid.blogspot.com



0 Response to "What Part Of The Atom Undergoes Change During Radioactive Decay"
Post a Comment